The basic form looks like this:
Let's go to the methods and examples.
PLUS
√4 + √3 = 2 + √3
MINUS
This is how we come up with 12 = 4 · 3 and 56 = 8 · 7.
The simplest way is to factorise the number within the last square root. Then find the ones which if added will produce the first number.
For instance √(8 + 2√15)
We have 8 as the first number and 15 as the last one.
Factors of 15:
- 1 · 15 = 15
- 3 · 5 = 15
Therefore, we choose the 2nd one, the 3 · 5 = 15
Because ➡️ 3 + 5 = 8
So we have x = 3 and y = 5
We put all together ➡️ √(8 + 2√15) = √3 + √5
Trick #1
Trick #2


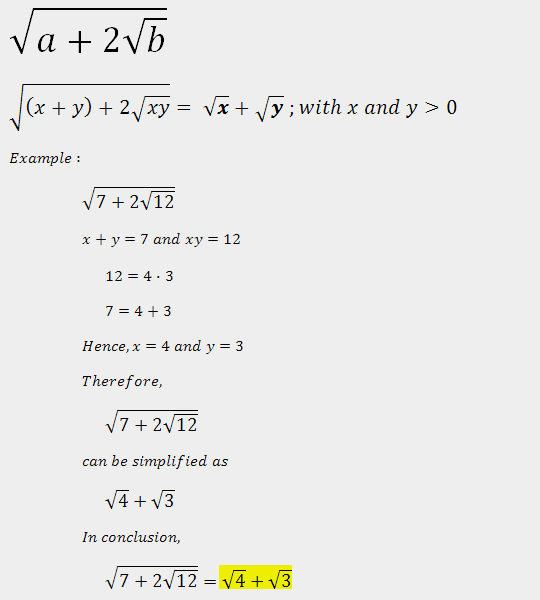



This was very useful. Thank you
ReplyDeleteYou're welcome.
DeleteWhat if the nested radicand doesnt have any coefficient.
ReplyDeleteWhat about that? Please be more specific by writing an example problem of that, or you could link an image/url.
DeleteThank you soo much, I have been looking for this solution for days!!!
ReplyDelete